How to Play Sudoku {rules & guide to solving a puzzle}

This post may contain affiliate links. This means Bailey’s Puzzles might receive a commission on the sale of certain items. This is at NO additional cost to you. Visit the policies page to learn more.

The Basic Sudoku Rules

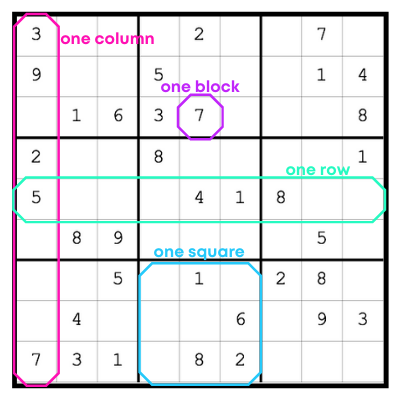
example of one block, square, column, and row for sudoku

A Sudoku game is played in a 9×9 grid.
Within the grid are 9 large squares (3×3 each), 9 rows (9×1 each), and 9 columns (1×9 each).
Each row, column, and square (of 9 blocks each) needs to contain the numbers 1 through 9 without repeating any numbers within that same row, column, or square.

Logic & Reason

Sudoku is not a guessing game!
Logic and reason should be used to determine the number that goes in each space.
I’ll be demonstrating how to solve a sudoku puzzle using this example:

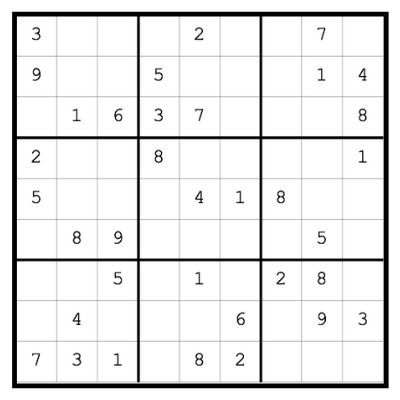
Solving Methods for Sudoku

There are several methods you can use to solve a sudoku puzzle.

Solving for Each Number

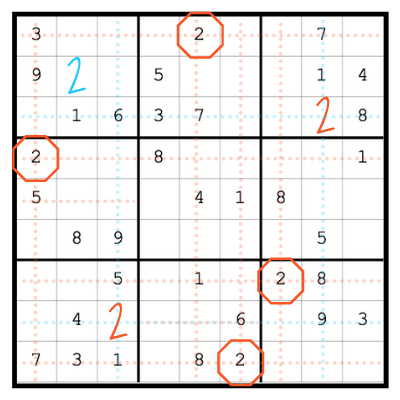
Start by choosing a number (I like to choose one that has a lot of the number on the board already).
Find all the numbers already given.
Eliminate everywhere that number cannot go.
Now, are there any obvious blocks you can fill in your number? If yes, do it!
If you add numbers, repeat steps 4 and 5. Once you don’t add anything, it’s time to move on.
Optional: mark any potential numbers (more on this next).

In this example, we’re going to try to fill out all the 2’s.

First, we found all the existing 2’s (circled in orange).
Then, we eliminated all the places a 2 cannot go (orange lines).
Next, we filled in two 2’s (orange, upper right and bottom left squares).
Then, we repeated in blue (which gave us one more 2).

Optional: Mark Potential Numbers

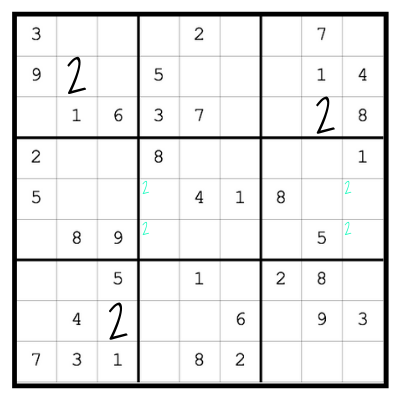
When you go through a number, you may want to mark off where that number could potentially go.
You can do this for any method of solving a sudoku puzzle.

For our example, we need two more 2’s and there are only 4 blocks that they could potentially go into. I’ve added small 2’s to the top of those boxes in green.

Solving by Square

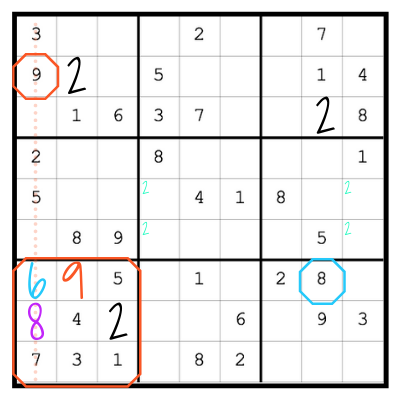
One method to solve a sudoku puzzle is to do it one square at a time.

Start by choosing a square (I like to choose one that only needs a few numbers).
Determine which numbers you need.
Look in the columns and rows for those numbers.
Fill out numbers where possible.
Once you can’t add any more numbers, it’s time to move on.
Optional: mark any potential numbers.

This is easier to see with an example. We’re going to do the bottom left square.

First, we selected the square (bottom-left).
Then we determined the missing numbers (6, 8, 9)
I started with the column furthest to the left.
- Does it have a 6, 8, or 9? YES, it has a 9 which means there is only one place I can put a 9 in my square. (orange)
There are no more columns I can check, so I’ll look in the top row next.
- Is there a 6 or an 8? YES! The top row has an 8, so my top block must have a 6. (blue)
This leaves me with only one free block in the square, which has to contain an 8. (purple)
This square is completely filled out! YAY!

Solving by Row

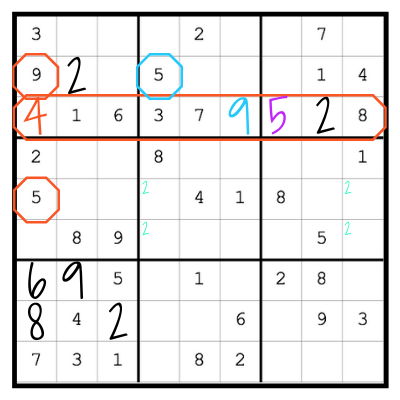
Another method when playing sudoku is to solve by row.

Start by choosing a row (I like to choose one that only needs a few numbers).
Determine which numbers you need.
Look in the columns and squares for those numbers.
Fill out numbers where possible.
Once you can’t add any more numbers, it’s time to move on.
Optional: mark any potential numbers.

Let’s check out our example. We’re going to do the third row from the top.

First, we selected the row (third from the top).
Then we determined the missing numbers (4, 5, 9)
I started with the column furthest to the left. Does it have a 4, 5, or 9?
- YES, it has a 5 and 9 which means the block must have a 4. (orange)
I’ll check the next column over that needs a number. Does the column have a 5 or 9?
- No. Does that square have a 5 or 9?
- Yes, it has a 5 so we’ll put a 9 in this block. (blue)
This leaves me with only one free block in the row, which has to contain a 5. (purple)
This row is completely filled out! YAY!

Solving by Column

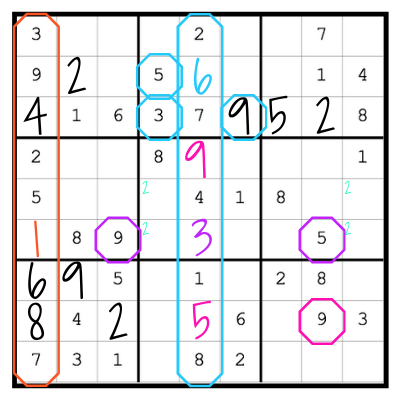
You can also try to solve a sudoku puzzle by column.

Start by choosing a column (I like to choose one that only needs a few numbers).
Determine which numbers you need.
Look in the rows and squares for those numbers.
Fill out numbers where possible.
Once you can’t add any more numbers, it’s time to move on.
Optional: mark any potential numbers.

Let’s check out our example. We’re going to do the column furthest to the left.

First, we selected the column (furthest to the left).
Then we determined the missing numbers (1)
There’s only one missing number, so we’ll just fill it out. (orange)

That was too easy! Let’s do another column example (the middle column).

First, we selected the column (middle column).
The we determined the missing numbers (3, 5, 6, 9)
I’m going to start from the top and work my way down, doing both the row and square at the same time.
The top empty block cannot have a 3, 5, or 9 so it must contain a 6. (blue)
The second empty block doesn’t have a 3, 5, or 9 in the square or in the row so it could be any of them.
The third empty block has a 5 and a 9 in the row, so it must be a 3. (purple)
The fourth empty block has a 9 in the row, so it must contain a 5. This means our second empty block must contain the 9. (pink)
This column is completely filled out!

Can You Finish the Example?

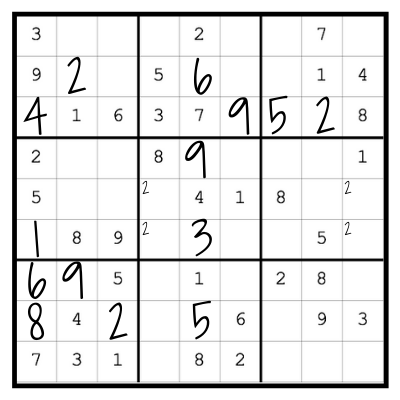
We’ve done a lot of our sudoku example. Try to finish it up yourself (click here for a printable version)!

You can use the number, square, row, or column method (or a combination of all the methods).

There’s One More Method

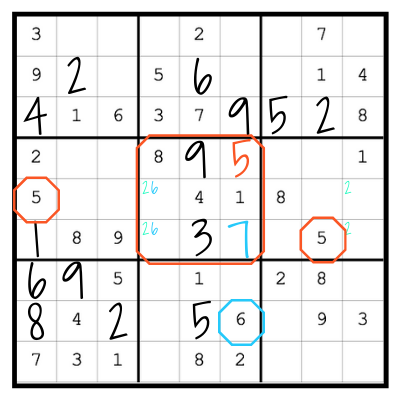
But what about those potential numbers you’ve been (optionally) marking? Let’s see how those can helpful by filling out the middle square.

First, we selected the square (center).
Then we determined the missing numbers (2, 5, 6, 7)
A quick look shows me that the bottom two rows of the square both contain a 5 which means our 5 must go in the top right block. (orange)
The right column cannot be a 6 (because there’s one in the column) or a 2 (because we’ve already marked any potential 2’s), so it must be 7. (blue)
Now we know that the remaining two boxes must be 2 and 6, but we don’t know which is which (yet).

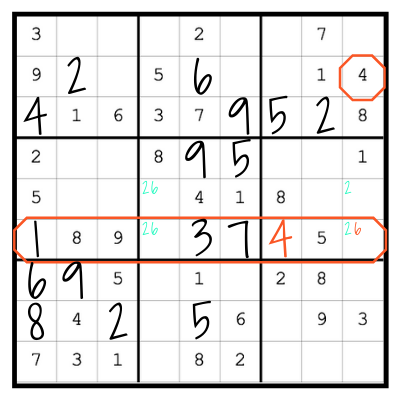
Let’s checkout the 6th row from the top (or 4th row from the bottom).

First, we selected the row (4th from the bottom).
Then we determined the missing numbers (2, 4, 6)
The furthest column contains a 4, which means that block must be a 2 or a 6. We also know that the blank left block must contain a 2 or a 6. This means the middle block can only be a 4.
The right column cannot be a 6 (because there’s one in the column) or a 2 (because we’ve already marked any potential 2’s), so it must be 7. (blue)
Now we know that the remaining two boxes must be 2 and 6, but we don’t know which is which (yet).

Let’s Finish the Example

I’m going to work (mostly) by square because that’s my preferred method. But, you could start wherever you wanted (by row, column, or number).

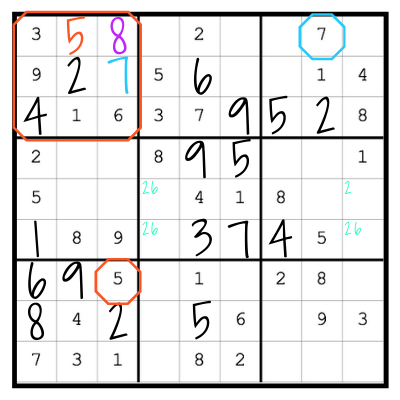
The Top-Left Square

Missing: 5, 7, 8
There’s a 5 in the right-most column, which leaves only one block free for a 5.
There’s a 7 in the top row, which leaves only one block free for a 7.
With the 5 and 7 filled out, we know where the 8 goes.

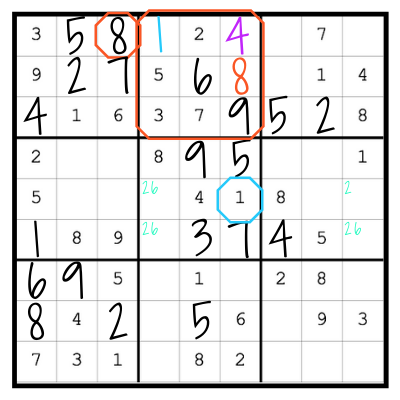
The Top-Middle Square

Missing: 1, 4, 8
There’s an 8 in the top row, which leaves only one block free for an 8.
There’s a 1 in the right column, which leaves only one block free for a 1.
With the 1 and 8 filled out, we know where the 4 goes.

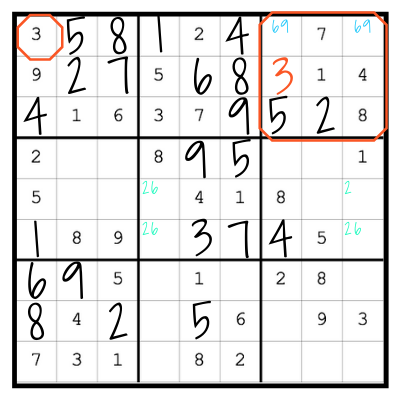
The Top-Right Square

Missing: 3, 6, 9
There’s a 3 in the top row, which leaves only one block free for a 3.
But, we don’t know where the 6 and 9 will go so we’ll mark them as potentials.

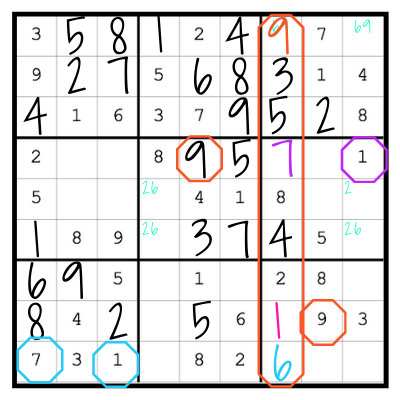
The Third-Column From the Right

Missing: 1, 6, 7, 9
There’s a 9 in the bottom square and a 9 in the middle row, so the only block that can be a 9 is the top one.
There’s a 1 and a 7 in the bottom row, so the block must be a 6. (blue)
There’s a 1 in the middle square, so that block must be a 7.
With the 6, and 7, and 9 filled out, we know where the 1 goes. (pink)

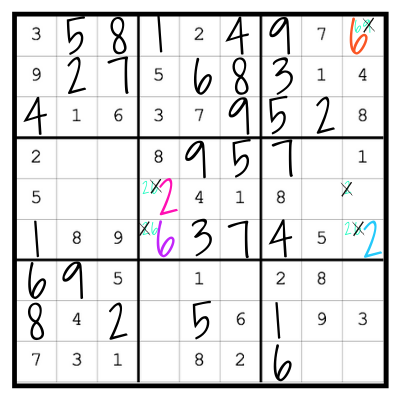
BONUS! The potential numbers we’ve marked down can be really helpful here.

Top-Right Square: We’ve filled out the 9, so we know the top corner block must be the 6. (orange)
Middle-Right Square: we see one block that must be a 2 or a 6. Since we’ve added a 6 to that column, it must be a 2. (blue)
Center Square: we know the bottom block must be a 6 (because we just filled out the 2 in this row). (purple)
Finally, we can fill out the remaining middle square block with a 2. (pink)

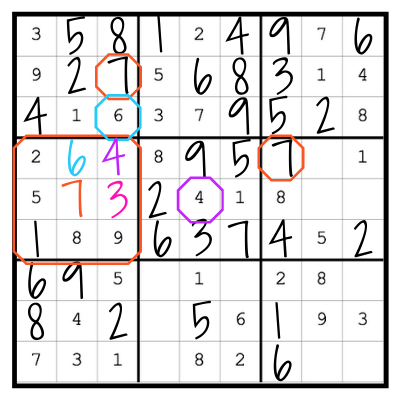
The Middle-Left Square

Missing: 3, 4, 6, 7
There’s a 7 in the top row and a 7 in the right column, which leaves only one block free for a 7. (orange)
There’s a 6 in the right column, which leaves only one block free for a 6. (blue).
There’s a 4 in the middle row, which leaves only one block free for a 4. (purple)
With the 4, 6, and 7 filled out, we know where the 3 goes. (pink)

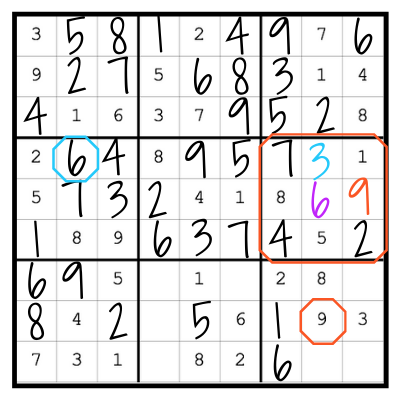
The Middle-Right Square

Missing: 3, 6, 9
There’s a 9 in the middle column, which leaves only one block free for a 9. (orange)
There’s a 6 in the top row, which leaves only one block free for a 6. (blue)
With the 6 and 9 filled out, we know where the 3 goes. (purple)

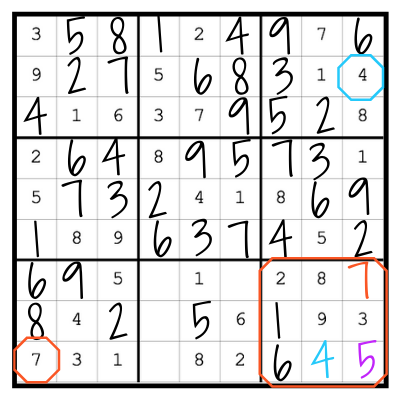
The Bottom-Right Square

Missing: 4, 5, 7
There’s a 7 in the bottom row, which leaves only one block free for a 7. (orange)
There’s a 4 in the right column, which leaves only one block free for a 4. (blue)
With the 4 and 7 filled out, we know where the 5 goes. (purple)

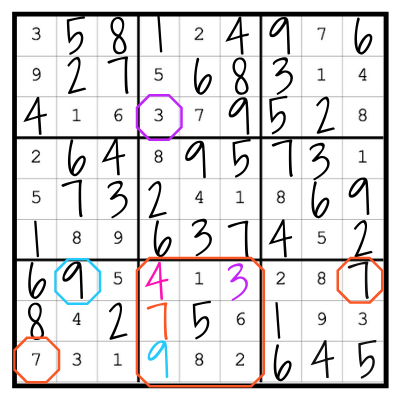
The Bottom-Middle Square (LAST ONE!)

Missing: 3, 4, 7, 9
There’s a 7 in the bottom row & top row, which leaves only one block free for a 7. (orange)
There’s a 9 in the top row, which leaves only one block free for a 9. (blue)
There’s a 3 in the left column, which leaves only one block free for a 3. (purple)
With the 3, 7, and 9 filled out, we know where the 4 goes. (pink)

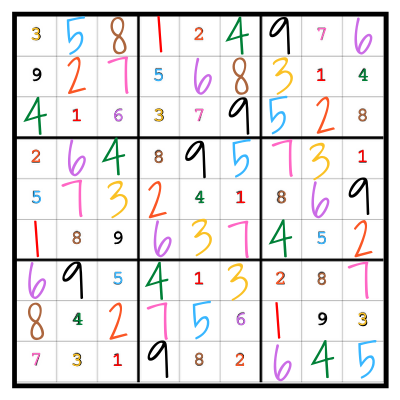
The Completed Sudoku Puzzle

I’ve given every number a unique color so that you can see the number patterns. Every single square has 1-9, every single row has 1-9, and every column has 1-9.